Fractions on a Number Line

One major misconception arises when students don't fully understand that fractions are unique numbers, like decimals, that occupy a specific place on the number line. Just like 1 fits nicely in a spot on the number line, so does 1/2.
Once students begin to see fractions as numbers, they are better equipped to understand that the same rules of addition, subtraction, multiplication, and division apply to fractions, just like all other numbers.
Here are two visuals that I have used with my students to help them visualize fractions on a number line. They are great additions to students' strategy notebooks or math journals:
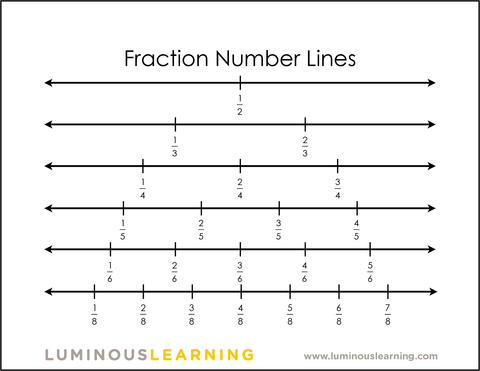
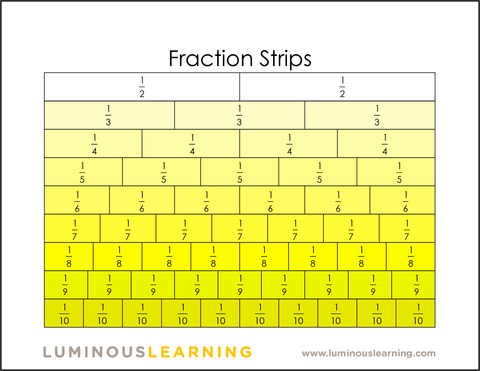
Here are some tips for teaching fractions:
1. To build students' flexible understanding of fractions, introduce them both as "how many" and "how much"
- How many: Use discrete objects. You have 5 marbles and 1 is red. What fraction of the marbles is red?
- How much: Use whole objects (pies, pizzas, chocolate bars, rectangles.) You divide a pie into four pieces. What fraction of the whole is one slice?
2. When using shapes such as rectangles to model fractions, identify the whole as the "area of the unit rectangle," not the rectangle itself. For example, don't say: "I'm dividing the rectangle into four equal parts." Instead, say, "I'm dividing the area of the unit rectangle into 4 equal parts." This prevents students from developing the misunderstanding that a fraction is a geometric object instead of a number.
3. Unit fractions have 1 as the numerator, such as 1/4. Larger fractions can be explained by combining unit fractions. For example, when introducing 3/4, explain and demonstrate that it's putting together 1/4 + 1/4 + 1/4. 3/4 is then understood to be 3 copies of the unit fraction 1/4. This will build a strong foundation for determining equivalent fractions and adding/subtracting fractions later on. The number line is a great tool to demonstrate this:

4. Equal fractions are called equivalent fractions. We should be careful not to define equivalent fractions as naming the same amount or being the same size because students might make the following incorrect assumption:

Instead, we can define equivalent fractions as two fractions that have the same value and occupy the same position on the number line.

The number line is also useful when comparing fractions. Students can be taught that a fraction to the left is smaller while a fraction to the right is larger, similar to how we use the number line to compare whole numbers.
What are some ways you use the number line to teach fractions in your classroom?

