Odd vs. Even Numbers

Learning the difference between odd and even involves more than just memorizing a string of numbers: 1, 3, 5, 7… vs. 2, 4, 6, 8, … Students should understand what it means to be even or odd:

Even Numbers: can be divided into two even groups with no remainders

Odd Numbers: when divided into two groups, there will always be one remainder

Here are two ways to help students understand what it means to be odd or even:

1. Use 10-frames: 

Students draw or place counters on the 10-frame to represent a number. They can describe the number as odd or even based on whether it "has a partner" or not.

(Download this FREE 10-frame printable here)

For example, the number 5 is odd because the last circle has not partner. I like to pretend the 10-frame is a bus and ask students, "Is there someone on the bus without a partner? Then it must be an odd number!"

You can also make a 12-frame out of old egg cartons. Students can place marbles or other small objects into the holes to represent numbers. 

2. Use the number line:

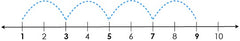
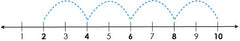
Have students trace the odd and even numbers on the number line. They can trace with their fingers, then a pencil, and then go over the pencil mark with a crayon for an extra kinesthetic experience. Afterwards, students should verbalize the pattern aloud or in writing. "The odd numbers skip over every other number, starting from 0. The even numbers also skip over every other number, starting from 2."

For these useful odd vs. even worksheets, check out our Grades K - 1 Number Sense and Place Value workbook.