What is an area model? 

An area model is a useful tool you can use to model certain fraction concepts. An area model is a square that you divide into equal-sized rectangles to represent a fraction. Here are some math concepts you can model with fraction sticks and area models:

Equivalent Fractions

A prevalent theme in the Grade 4 Common Core standards is understanding equivalent fractions, or, more precisely, the notion that a fraction remains the same when you multiply the numerator and denominator by a non-zero whole number. Understanding equivalent fractions is important when comparing and ordering fractions, adding and subtracting fractions with unlike denominators, and reducing fractions to their lowest term. So, let’s talk about finding equivalent fractions!

This is a great time for students to experiment informally with fraction sticks. They can choose a fraction, such as 2/3, and see what combinations of other fractions are equivalent, such as 8/12. After various opportunities to experiment informally with fraction sticks and write down their observations, they will be ready to learn a more formal rule: when you multiply the numerator and denominator by the same non-zero number, you will obtain an equivalent fraction. If your students are ready to be challenged with the symbolic form, you can explain:

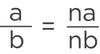
If we were to demonstrate 2/3 = 8/12 fact using an area model, first divide the area of the unit square into three rectangles. Shade in 2/3 of the area of the unit square.

Next, divide the area of the unit square into four horizontal rectangles (to demonstrate that you're multiplying both the numerator and denominator by 4). What is the new fraction represented by the shaded area? 8/12.

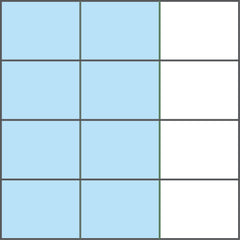
Multiplying Fractions

Let's take a look at a multiplication problem: 2/3 x 3/4. To demonstrate this with an area model, begin by dividing the unit square vertically into thirds. Shade in 2/3.

Next, divide the unit square horizontally into fourths. Shade in 3/4. 

What fraction is represented by the intersection of the two shaded areas? 6/12. In other words, 2/3 x 3/4 = 6/12