When teaching addition, it's important to ground students' understanding in the physical space. This helps build a conceptual basis for addition and helps them develop a spatial understanding of quantities. For example, how does 3 look and feel different than 10? When I add 3 + 10, how many more spaces do I need to advance relative to 3 + 2? Two useful tools when teaching addition are the number line and 10-frame. These types of visual representations not only build a conceptual understanding of addition, they also address a second goal: developing fact fluency. 

 

How do we use the number line and 10-frame to teach addition? Here are some ways to integrate these visual aids when teaching addition strategies: 

 

In this early stage of learning addition, a student will count all the numbers, starting with the first addend.

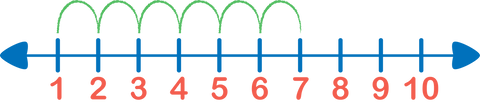
It sounds like: 1, 2, 3 … 1, 2, 3, 4 … 1, 2, 3, 4, 5, 6, 7 

It looks like: Demonstrate how you begin from 1 and hop all the way to 7. Count aloud as you "hop" the number line. 

At this stage, students are becoming more proficient with adding. Instead of starting with 1, they realize they can begin counting with the first addend.

It sounds like: 3, 4, 5, 6, 7. 

It looks like: Demonstrate how you begin from 3 and hop 4 more spaces. Count aloud as you "hop" the number line.  

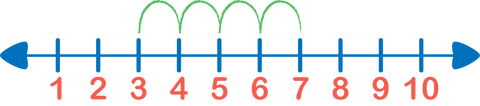
Instead of starting from the first addend, students begin to develop a more efficient method to add. They identify the larger addend and count on from there.

It sounds like: 4, 5, 6, 7. 

It looks like: Demonstrate how you identify the larger number (4) and begin counting on from 4, hopping 3 more spaces. Count aloud as you "hop" the number line.

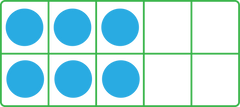
I like to use the 10-frame (or 20-frame, when moving into larger addition problems) when teaching the doubles. Let's take a different addition problem: 3 + 3. Students can visually see how 3 + 3 = 6, which reinforces the concept that the sum in all doubles problems will be even numbers because they have pairs. 

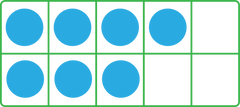
Once students demonstrate automaticity with their doubles facts, they're ready for doubles + 1. An example of a doubles +1 problem is 3 + 4. You can link this to a known doubles fact: 3 + 3 plus one more.

It sounds like: Demonstrate using a 10-frame how 3 + 4 is the same as 3 + 3 + 1. Once they recognize a known doubles fact, they can add one more to quickly calculate the sum. 

The 10-frame is the perfect tool to teach students how to create addition problems that equal 10. Students can experiment with different amounts, adding more circles until the 10-frame is filled up and recording their addition problems. 

It sounds like: Begin by giving students an amount: Here is the number 6. How many more circles do I need to fill up the whole 10-frame?

It looks like: 

One more useful tip: Whenever teaching this early addition strategies, I clap along as I recite the numbers aloud. For example, if we're adding 3 + 4, I'll start from the larger addend (4) and clap three times as I recite: 5, 6, 7. This provides an auditory component, helping students not only see how we add on the number line but hear the rhythm as they're adding. This auditory piece is very useful when we transition students from adding on paper to mental addition, as they need to recite the numbers in their minds as they add.

 

In addition to the number line and 10-frame, what are some useful visual tools you've used to teach addition?